Given a m * n matrix of ones and zeros, return how many square submatrices have all ones.

Example 1:

Input: matrix =
[
  [0,1,1,1],
  [1,1,1,1],
  [0,1,1,1]
]
Output: 15
Explanation: 
There are 10 squares of side 1.
There are 4 squares of side 2.
There is  1 square of side 3.
Total number of squares = 10 + 4 + 1 = 15.

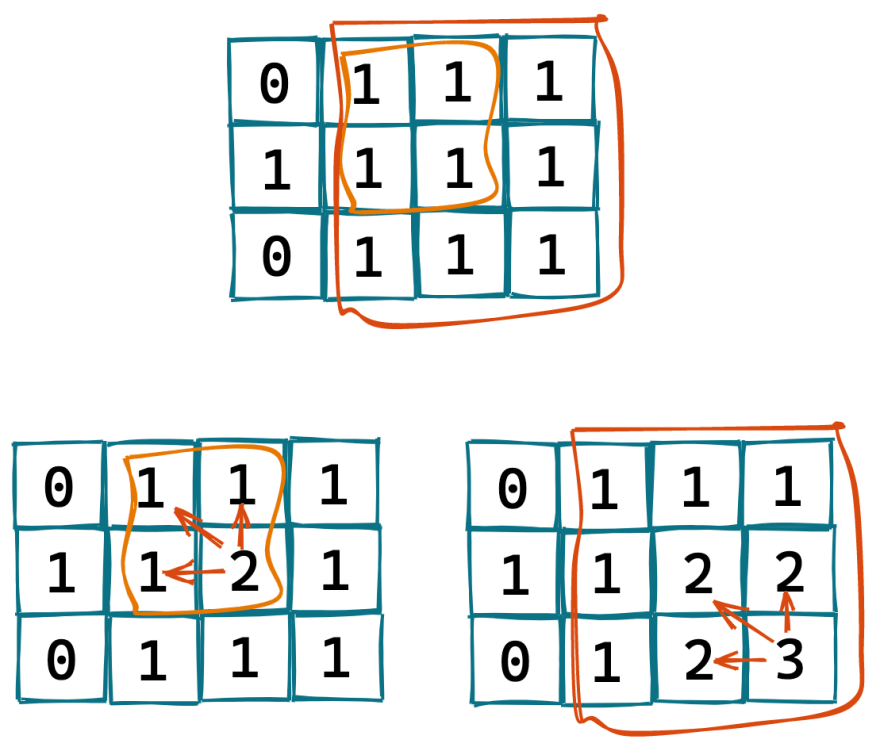
The trick here is to find how many squares can be formed at matrix[i][j]

From the image you can say that

matrix[i][j] = min(matrix[i - 1][j], matrix[i][j - 1], matrix[i - 1][j - 1]) + 1

In words it's the minimum of top, left and diagonal and the current square so add 1

You can solve this by recursion and checking matrix[i][j] can make a square, but it will be O(n^3)

To optimize we can use above technique, which is basically dynamic programming

Algorithm:

1. Initialize a result matrix to add up square matrices as encountered
2. Loop through each element matrix[i][j] and check the maximum square can be formed at that point
3. Add matrix[i][j] to res

Here's the code:

/**
 * @param {number[][]} matrix
 * @return {number}
 */
var countSquares = function(matrix) {
    let res = 0
    for(let i = 0; i < matrix.length; i++) {
        for(let j = 0; j < matrix[0].length; j++) {
            if(matrix[i][j] == 1 && i > 0 && j > 0) {
                matrix[i][j] = Math.min(matrix[i-1][j], matrix[i][j-1], matrix[i-1][j-1]) + 1
            }
            res += matrix[i][j]
        }
    }
    return res
};