There are multiple ways by which we can calculate the nth digit of pi by using Arctan formula and Bailey–Borwein–Plouffe formula.
Chudnovsky Algorithm is a fast way of calculating the digits of pi and is similar to the arctan's formula.This formula is derived from the Ramanujan’s π formulae.
Here's the Formula :-

Simplifying further by solving the Fractional Power:
Dividing the series further into two parts a and b we get:
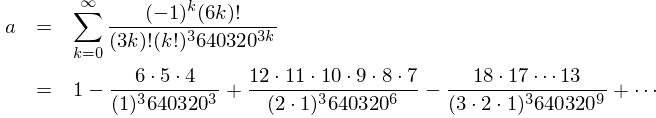
similarly,
we can calculate the next a term from the previous one, and the b terms from the a terms for simplifying the calculations.

Implementing the formula using Python
import math
def sqrt(n,m):
m1=10**16
m2=float((n*m1)//m)/m1
b=(int(m1*math.sqrt(m2))*m)//m1
n_m=n*m
while True:
a=b
b=(b+n_m//b)//2
if b==a:
break
return b
def power(n):
if n==0:
return 1
r=power(n//2)
if n%2==0:
return r*r
return r*r*10
def pi():
m = power(100000)
c = (640320**3)//24
n = 1
Ak = m
Asum = m
Bsum = 0
while Ak != 0 :
Ak *= -(6*n-5)*(2*n-1)*(6*n-1)
Ak //= n*n*n*c
Asum += Ak
Bsum += n * Ak
n = n + 1
result = (426880*sqrt(10005*m,m)*m)//(13591409*Asum+545140134*Bsum)
return result
stringPi = str(pi())
n = int(input())
print(stringPi[n-1])
This was the solution for GeeksForGeeks Problem.


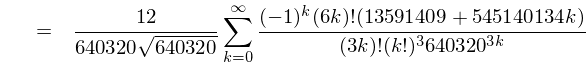


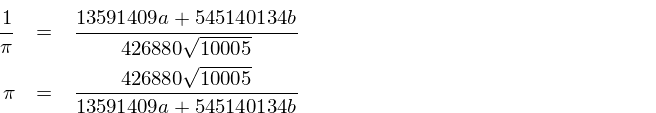





Top comments (0)